Trigonometry
-
guys, can help me with this?
sin(2x) - cos(x) = 1
answers are:
pi
4.357 154 322 516 74


-
wat u doing?
-
Paiseh hor, only can give partial answer. I think you need to find some software to compute the other answer.

sin 2x - cos x = 1 2 sin x cos x = 1 + cos x
2 sin x cos x = 1 + cos x
Square both sides and let y = cos x 2y^2 * (1 - y^2) = (1 + y)^2
2y^2 * (1 - y^2) = (1 + y)^2
Expand everything out: 4y^4 - 3y^2 + 2y + 1 = 0
4y^4 - 3y^2 + 2y + 1 = 0 (y + 1)(4y^3 - 4y^2 + y + 1) = 0
(y + 1)(4y^3 - 4y^2 + y + 1) = 0 y + 1 = 0 or 4y^3 - 4y^2 + y + 1 = 0
y + 1 = 0 or 4y^3 - 4y^2 + y + 1 = 0 y = - 1 or 4y^3 - 4y^2 + y + 1 = 0 (Have answer but I think y is an irrational number in this case)
y = - 1 or 4y^3 - 4y^2 + y + 1 = 0 (Have answer but I think y is an irrational number in this case) cos x = - 1 and the other answer
cos x = - 1 and the other answer x = Pi and the other answer.
x = Pi and the other answer.


-
as above

-
Easy to say hor?Originally posted by alexkusu:as above

-
Originally posted by CoolMyth:
got the same answer and working.
sin 2x - cos x = 1 2 sin x cos x = 1 + cos x
2 sin x cos x = 1 + cos x
Square both sides and let y = cos x 2y^2 * (1 - y^2) = (1 + y)^2
2y^2 * (1 - y^2) = (1 + y)^2
Expand everything out: 4y^4 - 3y^2 + 2y + 1 = 0
4y^4 - 3y^2 + 2y + 1 = 0 (y + 1)(4y^3 - 4y^2 + y + 1) = 0
(y + 1)(4y^3 - 4y^2 + y + 1) = 0 y + 1 = 0 or 4y^3 - 4y^2 + y + 1 = 0
y + 1 = 0 or 4y^3 - 4y^2 + y + 1 = 0 y = - 1 or 4y^3 - 4y^2 + y + 1 = 0 (Have answer but I think y is an irrational number in this case)
y = - 1 or 4y^3 - 4y^2 + y + 1 = 0 (Have answer but I think y is an irrational number in this case) cos x = - 1 and the other answer
cos x = - 1 and the other answer x = Pi and the other answer.
x = Pi and the other answer.
-
ok thank chiu~~ not allowed to compute as it is add maths level.Originally posted by CoolMyth:Paiseh hor, only can give partial answer. I think you need to find some software to compute the other answer.



the computation are as follows: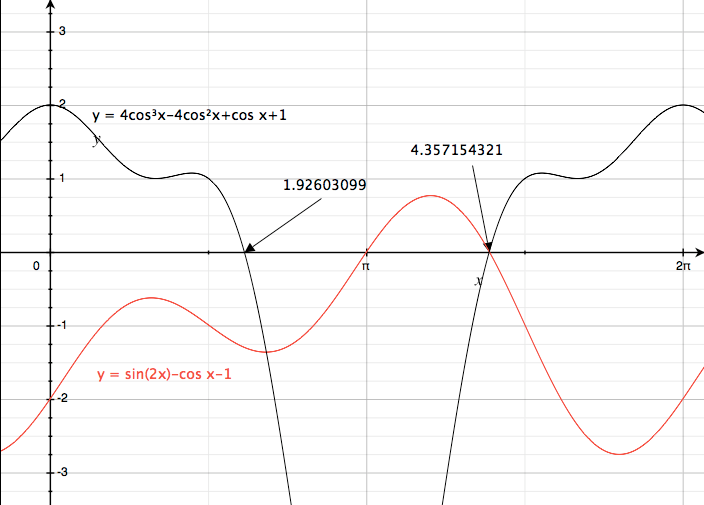
-
What is the range of your answer. From 0 to 2Pi?
Anyway, if you observe, that 1.9 something is also an answer. You go press calculator, you'll find that cos 1.9 something (in radian) = cos 4.3 something (in radian).
-
but the original equation, denoted by the red curve, does not have the 1.9 as one of its root.Originally posted by CoolMyth:What is the range of your answer. From 0 to 2Pi?
Anyway, if you observe, that 1.9 something is also an answer. You go press calculator, you'll find that cos 1.9 something (in radian) = cos 4.3 something (in radian).

-
kill me pls..



no maths....


-
as above.
-
If you use cos^-1 (1.926) = error because the peak and trough for cosine curve is from -1
So when you sub y=cos x, you must indicate y is -1<=y=<1. -
Originally posted by Gasfene:If you use cos^-1 (1.926) = error because the peak and trough for cosine curve is from -1
So when you sub y=cos x, you must indicate y is -1<=y=<1.


why u need to find acos(1.926)? we're trying to find cos(x), not acos(x). -
cos you sub in y =cos x (y can only be from -1 to 1)(remember cosine curve)Originally posted by ^tamago^:


why u need to find acos(1.926)? we're trying to find cos(x), not acos(x).
you get the answer for y=1.926
You try to get x from y, you cant get any x because cos^-1(1.926) is undefined. -
ahhhhhhhh!!! maths!!!! close n ban!



-
y=sin(2x)-cos(x)-1
min value: -2.760172593 at x=-0.634866875
max value: 0.760172593 at x=3.776459527 -
Why are you showing this?Originally posted by ^tamago^:y=sin(2x)-cos(x)-1
min value: -2.760172593 at x=-0.634866875
max value: 0.760172593 at x=3.776459527
We are looking for the root. not the min or max value. -
do u realise the 1.926 answer, the parameter used is cos(x) instead as shown in the graph?Originally posted by Gasfene:cos you sub in y =cos x (y can only be from -1 to 1)(remember cosine curve)
you get the answer for y=1.926
You try to get x from y, you cant get any x because cos^-1(1.926) is undefined.
it means the root is at cos(1.926) (which produces an answer where |cos x| <= 1) and not acos(1.926).
here i am solving for x directly, where x is of radian measure.
cos(x) where x is a of radiam/degree dimension
acos(x) where x is a real number of no dimensions
nothing to do with the subtituting y or not, which is another method. maybe u can use a = cos x in coolmyth's workings if u find y too confusing for u. -
cos y is not between -1 and 1.Originally posted by Gasfene:Why are you showing this?
We are looking for the root. not the min or max value. -
the y here i am using is the part where coolmyth used as substitution. And not the y in your equation.sin 2x - cos x = 1
2 sin x cos x = 1 + cos x
Square both sides and let y = cos x
2y^2 * (1 - y^2) = (1 + y)^2
[/quote]
The problem occurs here. the y must be between -1 to 1 because it is a cosine curve.
It is very hard to explain.
The other graph works because it isnt restricted to -1 to 1.
[quote]Originally posted by ^tamago^:cos y is not between -1 and 1. -
for ur y = cos x (written as x here), y = cos x = 0.3478448987 and x = 1.9260678004 or 4.357154319.
the second answer is actually within 99.92% of square root of 19. not sure if this is the result.
-
Errmmm.....getting too confusing for me. I think I'm lost.
Whatever lah.
-
dunno lah....i hate maths anyway
-
Copying from CoolMyth's solution:
sin 2x - cos x = 1
2 sin x cos x = 1 + cos x
Square both sides and let z = cos x (where z must be between -1 and 1 since it is a cosine curve)
4z^2 * (1 - z^2) = (1 + z)^2
Expand everything out:
4z^4 - 3z^2 + 2z + 1 = 0
(z + 1)(4z^3 - 4z^2 + z + 1) = 0
z + 1 = 0 or 4z^3 - 4z^2 + z + 1 = 0
z = - 1 or 4z^3 - 4z^2 + z + 1 = 0
z = - 1 or z = -0.3478448987
cos x = -1 or cos x = -0.3478448987
x = pi or x=1.9260678 + 2npi or x=4.357 +2npi for all n=integer.
Hi i am sorry about the earlier part. I wasnt addressing your problem. The only reason i can say is substitution can result in a lot of answer, of which only some of then is correct. -
Originally posted by Gasfene:
yes, but this is an add maths question.
Copying from CoolMyth's solution:
sin 2x - cos x = 1
2 sin x cos x = 1 + cos x
Square both sides and let z = cos x [b](where z must be between -1 and 1 since it is a cosine curve)
4z^2 * (1 - z^2) = (1 + z)^2
Expand everything out:
4z^4 - 3z^2 + 2z + 1 = 0
(z + 1)(4z^3 - 4z^2 + z + 1) = 0
z + 1 = 0 or 4z^3 - 4z^2 + z + 1 = 0
z = - 1 or 4z^3 - 4z^2 + z + 1 = 0
z = - 1 or z = -0.3478448987
cos x = -1 or cos x = -0.3478448987
x = pi or x=1.9260678 or x = 4.357 [/b]
how do u factorise to -0.3478448987 with a non-graphical calculator?
tat is the tricky part. or else why we need to think so long?
got graphical calc i also can find. on paper how? use newton-raphson?